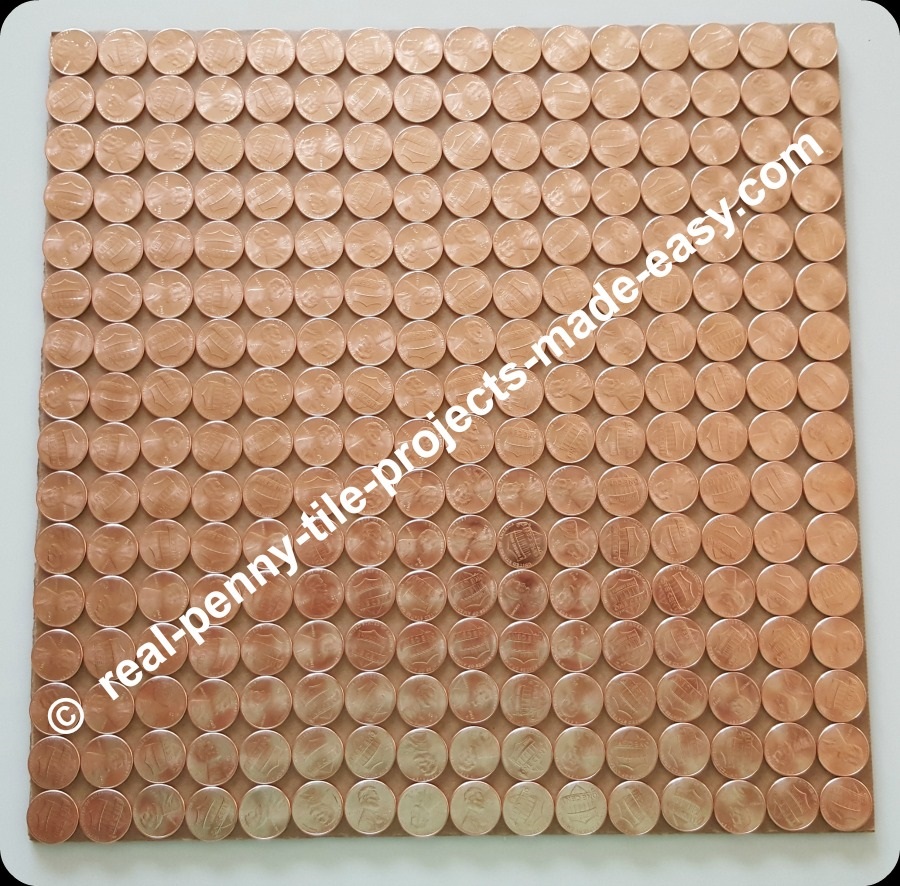
Tile Sheets of 224 Pennies Each  |  Real Penny Tile Projects Made Easy

How Many US Pennies Can Fit In One Square Foot (1 Sq. Ft/1 SF)

Before we find how many pennies can fit in one square foot, we must ask some questions...

• any minimum space required between pennies?
  
  
• what pattern?
  
  
• are there sides to the square foot and no penny can overlap any edge?
  
  
• other
  

Let's start with a piece of cardboard that measures 12 by 12 inches, which is exactly 1 SF. As you can see in the pictures, the tape measure shows we have 12 in. on both sides, even though it may be hard to see the small numbers.

We start placing pennies on the edge of this 12 in. cardboard and it results in a perfect fit: 16 pennies next to each other measure exactly 12 inches (one foot) because the diameter of a penny is .75 in. (or 3/4 of an inch).

By the way, as you can see, we used all brand new pennies for this demonstration.

We added 15 more pennies to 'fill' the other side of the square cardboard as you can see here:

Simple math can tell us how many pennies fit in one square foot in this straight pattern (16x16=256) without actually filling the square cardboard with pennies.


We decided to do it anyway in order to show other details and for you to see how beautiful it looks. Here we go, adding row after row of 16 pennies each. 

Worth mentioning here is that the pennies do touch each other. If a gap/space is required all around each penny, say for grout, changes must be made.   

So, how many pennies can fit in one square foot?
256 pennies per square foot if the rows are straight

No penny overlaps any edge and there's no space left whatsoever - at least that's what math tells us. If you see slight imperfections in the picture below is because we placed all 256 pennies by hand and they are just sitting there, not glued.

If a penny was square shaped instead of round, with a .75 in. side, the cardboard below would be completely covered by 256 square pennies. But because the penny is round, you can see the cardboard in between pennies.

Notice the empty area between any 4 pennies is quite big and has 4 rounded sides (more on this later).


After carefully placing every penny by hand, here it is: 
16 rows of 16 pennies each.

Here's a close-up on a corner of the square cardboard. Even though the pennies are in contact with each other, there's still quite some room in between them and that's due to the straight rows pattern/layout. 

And here's another view of the same 256 brand new shiny pennies sitting in straight rows on a precisely one square foot cardboard.

How many pennies can fit in 1 sq. ft. if we change the pattern to staggered/offset rows?

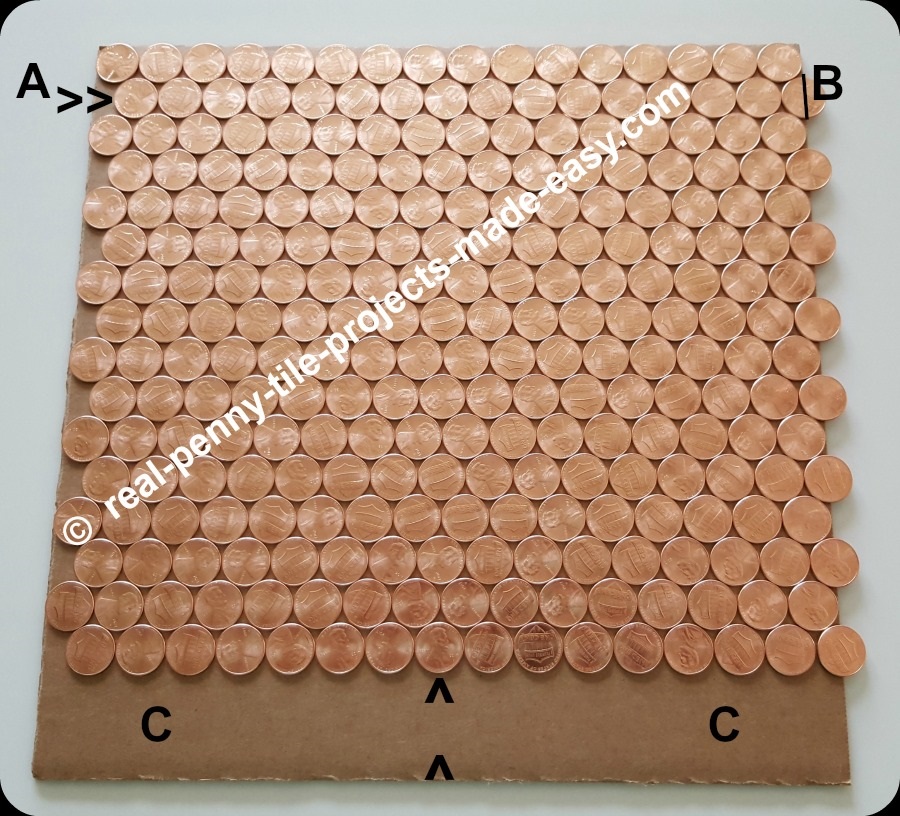
The first row of 16 pennies stays the same and we move the second row to the right by half a penny. Then we can also push up (as you look at the picture) the whole second row until it touches the first row of pennies.

We'll push every even numbered row (2nd, 4th, 6th, ...) to the right by half a penny and then the whole row up a bit to touch the previous row. With every row pushed up a little, we should gain some room at the bottom of the cardboard.

Here's more of a visual: Pushing a row by half a penny (A) results in half a penny overlapping the edge of our square foot (B). 

Overlapping can't happen if your precise one square foot (SF) project has sides/walls like a tray. And for bigger projects, each SF of pennies can overlap onto the next SF until you reach the end side of your project and cannot fit another full penny anymore.

So, pushing 8 rows to the right by half a penny and then up a little, our 16 original rows of pennies got 'squeezed upwards' closer together and revealed quite the extra room (C) at the bottom of the cardboard.

Can we fit 2 more rows of pennies there? We sure can. Not only that but there will be a bit of room left afterwards which we're not going to (mathematically) get into, but some more 'slices of pennies' will fit in the small room left below. 

Pennies per square foot (sf) in offset pattern:
288 plus about 6-8 pennies 'sliced' at top & bottom of cardboard
The total could be 294-296 pennies per SF

What if your perfect square foot was a tray (or similar) with edges/sides which don't allow pennies to overlap... like the 9 pennies do in the above or below picture.

So what then? Someone may say 'let's cut the 9 overlapping pennies in half and fill the left side with the 9 halves'. We totally advise against cutting pennies. Simply, not include the 9 overlapping pennies and the right side will be identical to the left.

Also, slide the whole thing down so the top and bottom will have identical spaces to the edge of the square foot.
Pennies per SF is 279 in this case (288-9=279).

Here's a close-up of the offset layout which brings up the subject of areas in between pennies. 

The straight pattern we saw earlier, had bigger empty areas with 4 rounded sides and now with the offset pattern we have smaller empty areas between pennies with 3 rounded sides. (Hey, where did one side go? Hmm...)

Let's compare the close-ups of both layouts one after the other. You can clearly see the pennies much closer together with smaller empty areas in the offset layout compared to the straight rows pattern with bigger empty areas in between the pennies. 

Remember that in both patterns the pennies are still touching each other and if grout is desired for your project, it's best to have a space all around each penny.


To sum up, here's the comparison of each penny pattern on a square foot cardboard, hoping it brings some light to...
 

How Many Pennies Fit In 1 SF

In both patterns above,
the pennies are touching each other.

What if a space/gap is needed all around each penny?

If you need the pennies to have a space for grout or other reasons, you most likely need our Real Penny Tile Sheets (handmade mesh-backed tile sheets of pennies).

Our handmade sheets of pennies have 224 pennies each. There are small gaps in between the pennies as room for grout (get 20% OFF at The Tile Shop for grout & tile). 

The whole sheet is slightly less than 1 SF in order to fit in shipping boxes that are 12 by 12 inches.

If you're a math genius and want to tackle the '32 slices article', please let us know and we'll publish it here with all due credit.

How can we help?

^Back to TOP^ of this page: How many pennies can fit in one square foot (1SF)

Go to Home Page: Tile Sheets Of Real Pennies - Real Penny Coin Tile